Calculating the stability of your equipment

Many components in a manufacturing environment must be secured to a cart or mount for proper functionality. The design process for this assembly, however, requires more than simply creating a mount. You must secure all key components and ensure the assembly is stable enough for all possible orientations and functional movements. This verification is crucial to protecting not only the equipment but also any nearby workers.

In this article, we’ll cover how to verify the stability of an assembly where the mounted equipment does not move while in use.

Stability Overview

The stability of an object generally depends on two things: the width of its base, and the height of its center of mass. We know intuitively that a narrow, top-heavy object is much less stable (i.e., easier to knock over) than a very wide, bottom-heavy object. The same principle must be kept in mind when designing mounting equipment. For example, if a certain application demands a pedestal-style mount, there must be extra weight near the base to balance out the weight at the top. On the other hand, it’s not so important to add weight near the base of the assembly is already quite wide.

The calculation procedure below further illustrates these concepts. For more advice and project-specific insights, ask the Vention Applications Engineering team for a Design Review.

Calculations

You must consider the weight of each component and the external force acting upon them (gravity). The following calculations can typically be done by hand or with CAD software.

To determine stability:

1. Calculate the center of mass of the overall assembly including all external loads.
2. Draw a cone (or the equivalent triangle, if working in 2D) with a) a half-angle of 18 degrees, and b) a tip placed at the overall center of mass.
3. Draw a circle (or line) where this cone or triangle intersects with the surface on which the assembly rests (usually the ground/floor).
4. Evaluate whether every one of the assembly’s points of contact is outside the circle or line above. If yes, the assembly is stable for static applications.

To see the principles in action, check out the example below:

Step 1: Calculate the cart’s center of mass (including any attached equipment).

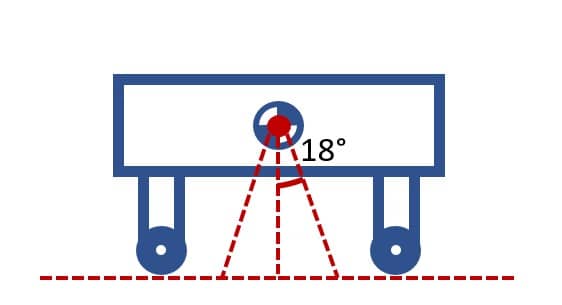
Step 2: Draw a triangle to assess the placement of the cart’s points of contact. Since they all fall outside the triangle, this cart is stable.

As you can see from these sketches, the assembly needs a pretty large base in order to be stable!

Conclusion

Equipment stability must not be overlooked. As highlighted above, guaranteeing your assembly won’t tip over can be achieved with this simple calculation process.

Have any questions? Our dedicated team of application engineers is here to help. Ask them about how they advise our users on stability and other key design issues. Talk to an expert today.